Here we've combined all the pieces from the three individual Tiny Tans into one large octagon that fits neatly into a 7" framed octagonal tray. Each of the three colors belongs to a different 4-piece puzzle, so you can easily separate them again to solve their individual challenges. With this Trio in a Tray, we include all three of their pattern cards, plus an extra one for combined figures (shown below).

Embedded shapes
An exceptionally interesting challenge is to embed in the tray one of the figures from any of the three pattern cards and fit all the other pieces around it. Not all figures are solvable this way. The solvable ones are marked with green on the three panels below. How many of them can you solve? Here are a few samples:

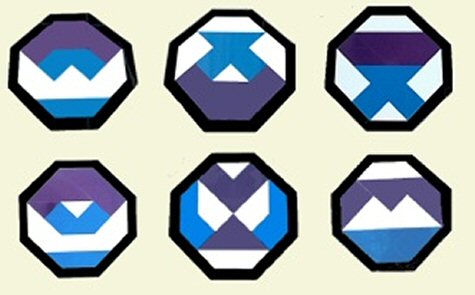
Figures and symmetries
Other interesting visual effects are just waiting to be discovered. Here are some by Kate Jones, Eric Bare and Meshele Merchant, with figures, mirror symmetry and opposite-color symmetry:

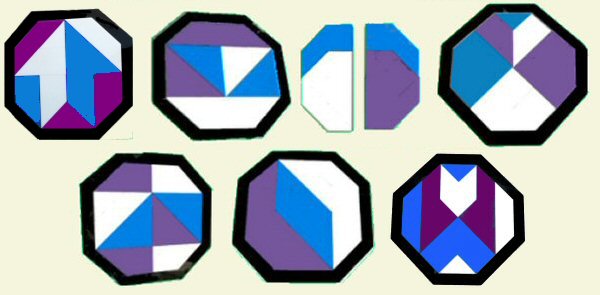
Color separations
Another theme asks for no two of the same color to share sides. It soon became evident that a color-separated solution cannot be symmetrical. These two are close, and they have the additional feature that one color doesn't even touch at corners.

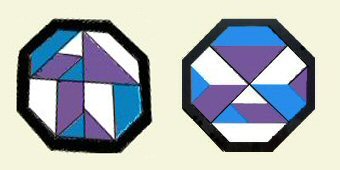
Color and shape separations
The solution in the tray at the top of this page has some other special properties:   no two pieces of the same color share sides (corners are okay), and no two of the same shape are joined.

Jeffrey Blitt found the second-ever shape- and color-separation solution on May 22, 2015, at our show in Montauk, NY, and won a prize. Here he is with his remarkable, nearly fully symmetrical pattern:

Photo by Meshele Merchant

 
On October 5, 2015, during a drizzly slow day at Ye Olde Gamery at the Maryland Renaissance Festival, our own stalwart and determined crew member (and game inventor), Art Blumberg, found the third-ever full separation of shapes and colors, and with a neat square in the middle. Notice the antique tablecloth, part of the Gamery's 16th century decor. A little later, Art found three more of these rare beasties, shown below.

 
In December 2016, Alex Streif was the fourth solver to find a new full separation of colors and shapes. Alex was Kadon's newest associate during 2017 before returning to his native Minnesota. His many talents supported our research, promotion and production efforts.

 
Along the way this separation solution turned up, making eight in all.

 
In 2019, Elijah Allen and Krystal Goodrich worked out these additional rare solutions (first row) with both colors and shapes separated; each can have minor variants. Elijah hunted down three more solutions (second row), bringing the total found to 13. The hunt continues.

 
Full color separation
Any one of the three colors can have its four pieces separated completely, not even corners touching. One solution for each is shown here:

Fully separating two colors simultaneously eluded several solvers until the end of November 2016, when Kate finally found one solution (left). Here it is with its minute variant (center). In October 2017, Kate found a second one (right), and the search goes on. Can the last pair of colors be separated? Find it or prove it impossible, and win a prize!

 
The "Trio-in-a-Tray" collection

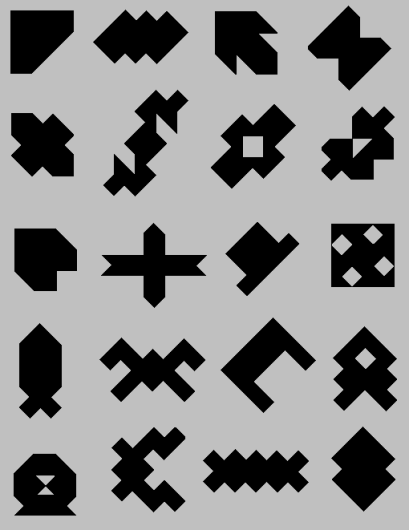
The group of silhouettes above is just a starter collection of the many symmetrical shapes you can form with all 12 pieces. There are hundreds of other shapes you can make, not using the tray. Be creative. Just remember always to join pieces according to their corresponding lengths of side, as explained for the individual Tiny Tans. Then see if you can solve the figures with color or shape separation, or both of those added conditions. We don't know how many of them are possible—send us your best results.

 
To order this Trio-in-a-Tray edition of Tiny Tans, just click the green logo below.   $42